Solving the Quadratic Equation: 4x² + 5x – 12 = 0

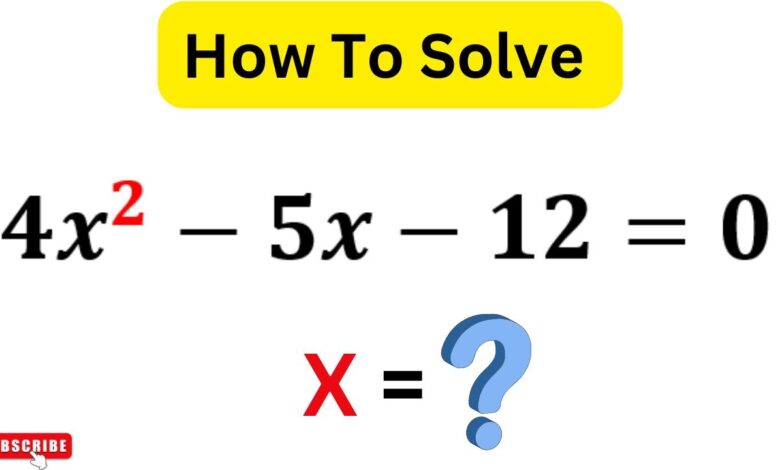
Quadratic equations are a fundamental component of algebra and are used to describe a wide range of real-world phenomena. One such equation is 4x² + 5x – 12 = 0. In this article, we will explore the process of solving this quadratic equation step by step, employing various methods to find the roots of the equation. Understanding these methods not only enhances your algebraic skills but also provides a deeper insight into mathematical problem-solving.

Understanding Quadratic Equations

A quadratic equation is a second-degree polynomial equation in a single variable x, with the standard form:

𝑎𝑥2+𝑏𝑥+𝑐=0ax2+bx+c=0

In our case, the quadratic equation is:

4𝑥2+5𝑥−12=04x2+5x−12=0

Here, $a=4$, $b=5$, and $c=-12$.

Methods to Solve the Quadratic Equation

There are several methods to solve quadratic equations, including:

1. Factoring
2. Using the Quadratic Formula
3. Completing the Square
4. Graphical Method

We will focus on the first two methods for solving 4x² + 5x – 12 = 0.

Method 1: Factoring

Factoring involves expressing the quadratic equation as a product of two binomials. For the equation 4x² + 5x – 12 = 0, we look for two numbers that multiply to $ac$ (where $a=4$ and $c=-12$) and add up to $b$ (which is 5).

First, calculate $ac$:

$ac=4\times -12=-48$

We need two numbers that multiply to -48 and add up to 5. These numbers are 8 and -6 because:

$8\times -6=-48$ $8+(-6)=2$

Now, rewrite the middle term (5x) using 8 and -6:

4𝑥2+8𝑥−6𝑥−12=04x2+8x−6x−12=0

Next, group the terms and factor each group:

$4x(x+2)-6(x+2)=0$

Factor out the common binomial (x + 2):

$(4x-6)(x+2)=0$

To solve for x, set each factor equal to zero:

$4x-6=0\text{or}x+2=0$

Solving these equations gives:

$4x-6=0$ $4x=6$ 𝑥=64x=46​ 𝑥=32x=23​

$x+2=0$ $x=-2$

Thus, the solutions to the equation 4x² + 5x – 12 = 0 are:

𝑥=32and𝑥=−2x=23​andx=−2

Method 2: Using the Quadratic Formula

The quadratic formula is a universal method for solving any quadratic equation and is given by:

𝑥=−𝑏±𝑏2−4𝑎𝑐2𝑎x=2a−b±b2−4ac​​

For our equation, $a=4$, $b=5$, and $c=-12$. Plugging these values into the quadratic formula:

𝑥=−5±52−4⋅4⋅(−12)2⋅4x=2⋅4−5±52−4⋅4⋅(−12)​​

Calculate the discriminant ($\Delta$):

Δ=𝑏2−4𝑎𝑐Δ=b2−4ac Δ=52−4⋅4⋅(−12)Δ=52−4⋅4⋅(−12) $\Delta =25+192$ $\Delta =217$

Now, find the roots:

𝑥=−5±2178x=8−5±217​​

So, the solutions are:

𝑥=−5+2178and𝑥=−5−2178x=8−5+217​​andx=8−5−217​​

Conclusion

Solving the quadratic equation 4x² + 5x – 12 = 0 reveals two distinct methods: factoring and using the quadratic formula. Both methods lead to the same solutions, although the quadratic formula provides an exact form that can be simplified further if needed. Understanding these techniques not only aids in solving specific problems but also enhances overall mathematical proficiency. Whether for academic purposes or real-world applications, mastering quadratic equations is an essential skill in the mathematical toolkit.